Precalculus Homework Unit 6 - chapter 5

Precalculus Homework Unit 6 - Chapter 5

5.1 Homework #38; Pg.381; 5, 7, 25, 27, 37, 43, 55, 59,
67, 71, 75-77odd, 91, 101, 103

5.2 Homework #39; Pg.389; 5-9odd, 21-25odd, 34, 35, 43, 55, 71;
*Pg. 381; 39, 41, 57, 69, 73, 93

5.3a Homework #40; Pg.400; 3, 11, 15, 23, 31, 39, 43, 47, 51, 55
*Pg. 389; 3, 19, 27, 37, 49, 59
Quiz on Sections 5.1-5.2 Next Class

5.3b Homework #41; Pg.400; 5, 7, 19, 27, 35, 41, 45, 53, 57, 59, 75, 76

5.4 Homework #42; Pg.408; 3, 5, 11, 15, 19, 25-27odd, 37-41odd, 45, 47 – 55 odd
*Pg 400; 7, 19, 27, 35, 43

5.5a Homework #43; Pg.418; 5, 7, 17, 19, 21-23odd, 31, 39,
43, 47, 51-57odd, *Pg 408; 21, 23, 35, 43, 57
Quiz on Sections 5.3-5.4 Next Class

5.5b Homework #44; Pg.418; 9, 18, 22, 27, 35, 45, 65, 71,
83-87odd, 103

Review Homework #45; Pg.422; 1 – 107 every 4th odd (1, 5, 9, …)

Homework #46; Chapter 5 Test

Precalculus 2.6 Rational Functions and Asymptotes

2.6 Rational Functions and Asymptotes

A rational function can be written in the form:

f(x) = N(x)/D(x)

where N(x) is the numerator and
D(x) is the denominator and both are polynomials but D(x) is not the zero polynomial (because you cannot divide by zero)

I. Asymptotes of a Rational Function:
Let "f" be the rational function f(x) where

f(x) = N(x)/D(x) where

N(x) = a_nxⁿ + a_n-1x^n-1 + ... + a₁x + a₀ and

D(x) = b_mx^m + b_m-1x^m-1 + ... + b₁x + b₀

Where N(x) and D(x) have no common factors.
1. The graph of "f" has vertical asymptotes at the zeros of D(x).
2. The graph of "f" has at most one horizontal asymptote determined by comparing the degrees of N(x) and D(x).
a. If n is less than m, the line y = 0 (x-axis) is a horizontal asymptote.
b. If n = m, the line y = (a_n)/(b_m) is a horizontal asymptote.
c. If n is greater than m, the graph of "f" has no horizontal asymptote.

Examples:
a) f(x) = (5x² + 3)/(-6x³ + 2x + 4)

n = 2 and m = 3 which means that n is less than m so the horizontal asymptote is y = 0

b) f(x) = (2x³ + 2x²)/(3x³ + 4x)

n = 3 and m = 3 so the line y = 2/3 is a horizontal asymptote.

c) f (x) = (3x⁴ + 2x² + 5)/(4x³ + 3x)

n = 4 and m = 3 so n is less than m, therefore there is not a horizontal asymptote.

II. Find the Domain and Asymptotes of f(x) = 3/ ((x - 2)³)

1. Find the vertical asymptotes by taking D(x) and setting it equal to zero.

(x - 2)³ = 0
x - 2 = 0
x = 2

therefore a vertical asymptote is the line x = 2

2. Find the horizontal asymptotes
n = 0 and m = 3 so n is less than m, therefore the line y = 0 is the horizontal asymptote.

3. Graph it using a graphing utility.

4. Domain (- ∞, 2) U (2, ∞)

5. Range (- ∞, 0) U (0, ∞)

6. check using a table:

{ (-3, -0.24), (-2, -.047), (-1, -.11), (0, -3.75), (1, -3), (2, error), (3, 3)}

Homework: Quiz (19, 20)
Pg. 187/ 7, 21, 23, 43*Pg. 195/ 13 – 19 odd, 27 – 31 odd, 35

Precalculus 2.5 The Fundamental Theorem of Algebra

2.5 The Fundamental Theorem of Algebra –
Proved by Carl Friedrich Gauss

If f (x) is a polynomial of a degree “n”, where n is greater than 0,
“ f ” has at least one zero in the complex number system.

I. Linear Factorization Theorem:
If f(x) is a polynomial of degree “n” where “n is greater than zero”, “f” has precisely “n” linear factors.

f (x) = a_n (x – c₁)( x – c₂)( x – c₃) … ( x – c_n) where
c₁, c₂, c₃, … c_n are complex numbers.

Factors of a Polynomial:

Example 1: f (x) = t⁴ - 5t³ + 15t² - 45t + 54

4th degree n = 4, n is greater than zero is true

Step 1: find all the possible zeros of the function:
(factors of 54)/(factors of 1) = ±1, ± 2, ± 3, ±6, ± 9, ± 18, ± 27, ± 54

Step 2: Use synthetic division to find out which ones are factors


f (x) = (x – 2)(x – 3)(x² + 9)
0 = (x – 2)(x – 3)(x² + 9)

0 = x – 2
x = 2

0 = x – 3
x = 3

0 = x² + 9
- 9 = x²
±√(-9) = x
± 3i = x

So to write this function in linear form:

f(x) = (x – 2)(x – 3)(x + 3i)(x – 3i)

So the following zeros of “f” are:

x = {2, 3, 3i, and -3i}

II. Complex Zeros Occur in Conjugate Pairs

In the last example, “± 3i”, the pair is 3i and -3i so we can conclude:

Let f(x) be a polynomial function that has real coefficients.
If a + bi, where b ≠ 0, is a zero of the function,
the conjugate a – bi is also a zero of the function.

Example: Your zeros are {2, 4 + i, and 4 – i}, find the polynomial function:

f(x) = (x – 2)(x – (4 + i))(x – (4 – i))
= (x – 2)((x – 4) – i)((x – 4) + i)
= (x – 2)( (x – 4)² - (i)²)
= (x – 2)(x² - 8x + 16 – (-1))
= (x – 2)(x² - 8x + 17)
= x³ – 8x² + 17x – 2x² + 16x – 34
= x³ – 10x² + 33x – 34

III. If you are given one zero, can you find the rest?

g(x) = 4x³ + 23x² + 34x – 10 given zero: -3 + i

Recall both conjugates:
– 3 + i and – 3 – i so


(x + 3 – i) (x + 3 + i)
= ((x + 3) – i)((x + 3) + i)
= ((x + 3)² - (i)²)
= x² + 6x + 9 – (-1)
= x² + 6x + 10

So using long division:


Every polynomial of degree n is greater than zero with real coefficients can be written as the product of linear and quadratic factors with real coefficients, where the quadratic factors have no real zeros.

A quadratic factor with no real zeros is said to be irreducible over the real numbers.

x² + 1 = (x + i)(x – i) is irreducible over the real numbers
x² - 2 = (x + √2)(x - √2) is irreducible over the rational numbers but reducible over the real numbers.

Example: The complex number 4i is a zero of f(x) = x⁴ + 13x² - 48.

Find the remaining zeros of f(x), and write it in its linear factorization.

Since 4i is a zero, then -4i is also a zero so

(x-4i)(x+4i) = x² - (4i)²

= x² - 16i²

= x² + 16

therefore a factor of f(x) is x² + 16

f(x) = x⁴ + 13x² - 48
= (x² + 16)(x² - 3)
= (x + 4i)(x – 4i)(x + √3)(x - √3)

So the zeros are:
x = {-4i, 4i, -√3, √3}

Homework: Pg. 170/ 57, 65, 69
*Pg. 187/ 1 – 5odd, 9 - 19odd, 33, 37, 41, 45, 53, 55, 61, 65

Precalculus 2.4 Complex Numbers

2.4 Complex Numbers

I. A complex number is written in standard form: a + bi
where a ε set of real numbers
and
bi is a pure imaginary number

Therefore a + bi is an imaginary number

√(-1) = i
(√(-1))² = i² = -1
(√(-1))³ = i³ = -i
(√(-1))⁴ = (√(-1))²(√(-1))² = (-1)(-1) = 1
(√(-1))⁰ = 1

Therefore you can see the pattern that:

i⁰ = 1
i ¹= √(-1) = i
i² = -1
i³ = -i
i⁴ = 1
so i⁵ = i
i⁶ = -1
i⁷ = - i
i⁸ = 1

so what would i⁶³ = ?

i⁶⁰i³
i⁶⁰ = 1 and so therefore
i⁶⁰i³ = (1) i³ = - i

B. Additive Identity is zero
what do you add to a number to get that number? of course, zero so

a + bi + ________ = 0

______ = -a - bi

C. Adding and Subtracting complex Numbers:

Example 1: (3 + 4i) + (7 + 2i)
= (3 + 7) + (4i + 2i) by grouping like terms
= 10 = 6i

Example 2: (3 + 4i) - (7 + 2i)
= (3 - 7) + (4i - 2i)
= (-4) + (2i)
= -4 + 2i

D. Multiplying Complex Numbers
Example 1: (3 + 4i)(7 + 2i)
= (3)(7) + (3)(2i) + (4i)(7) + (4i)(2i)
= 21 + 6i + 28i + 8i²
= 21 + 34i + 8(-1)
= 21 + 34i - 8
= 13 + 34i

E. Dividing Complex Numbers - multiply by the complex conjugate

Given "a + bi", the complex conjugate would be "a - bi"

Example 1: complex #1

but recall that "a + bi" has to be in standard form so...

29/53 + 22i/53 would be the answer!

F. Applications:
1. Fractal Geometry

Mandelbrot Set: to draw this, consider: Sequence of numbers

c, c² + c, (c² + c)² + c, [(c² + c)²+ c ]² + c, ...

For some values, it is Bounded, which means that all elements in the sequence are less than some fixed number N. Therefore, complex number "c" is in the Mandelbrot Set.

For other values, it is Unbounded, which means that the elements in the sequence become infinitely large. Therefore, complex number "c" is not in the Mandelbrot Set.

Check out this website:

http://mathworld.wolfram.com/MandelbrotSet.html

Example 1: Let's let c = -1

So the sequence would be:

-1, (-1)² + (-1) = 0, (0)² + (-1) = -1, (-1)² + (-1) = 0

or -1, 0, -1, 0

so this is bounded!

Example 2: Let's let c = - i

-i, (-i)² + (-i) = -1 + -i, (-1 -i)² + (-i) = -3i, (-3i)² + (-i) = -9 - i

or -i, -1 - i, -3i, - 9 - i

so this is not bounded.

2. Impedance - the opposition to current in an electrical circuit.

Equation of 2 pathways:

1/z = 1/z₁ + 1/z₂

where

z₁ is the impedance of pathway 1

z₂ is the impedance of pathway 2

Precalculus 2.3 Real Zeros of Polynomial Functions

Precalculus 2.3a

I. Long Division of Polynomials:

A. Recall: When you did long division

Example 1:

625/5 =

As you can see, 5 goes into 625 perfectly and there is no remainder so this means that 5 is a factor of 625.

Example 2:
625/4 =


As you can see, 4 does not go into 625 perfectly, there is a remainder of 1 so we would write the answer like

156 + 1/4

You write the answer or quotient plus the remainder over the divisor

B. Long Division of Polynomials:

Now try doing the same concept only using Polynomials:

Example 3:


So therefore since (x - 4) is a factor so

x - 4 = 0
x = 4

Therefore (x - 4) is a factor and since there is not a remainder, then (x - h) is a factor and x = h.

Example 4:

Therefore (x + 2) is a factor and since there is not a remainder, then (x - h) is a factor and x = h,
then x = -2

Example 5:




Since when you divide by (x + 1), it has a remainder, it is not a factor and x = -1 is not a zero.

Example 6:





So again, since (x - 2) is not a factor, x = 2 is not a zero.

Precalculus 2.3b Synthetic Division:
To divide ax³ + bx² + cx + d by (x - k), use the following pattern:

Example 1: (5x² - 17x - 12) / (x - 4)

Therefore, (5x² - 17x - 12) /(x - 4) = 5x + 3

since there is not a remainder!

Example 2: (x⁴ + 5x³ + 6x² - x - 2) / (x + 2)

Again, since the remainder is 0,

(x⁴ + 5x³ + 6x² - x - 2) / (x + 2) =

x³ + 3x² - 1

Example 3: (4x³ - 13x + 10) / (x + 1)

Since there is not an x², we put zero in that place:

Since 19 is the remainder:

(4x³ - 13 x + 10) / (x + 1) = 4x² - 4x - 9 + 19/(x + 1)

C. The Remainder Theorem: If a polynomial f (x) is divided by (x - k), the remainder is r = f (x)

Recall from Example 3, we saw the remainder = 19 so...

f (-1) = 4(-1)³ - 13 (-1) + 10= 4 (-1) + 13 + 10= -4 + 13 + 10= 19

this gives the same answer!!

Let's try another:

Example 4: (4x³ - 13x + 10) / (x - 2)

f(2) = 4(2)³ - 13(2) + 10 = 16, again, the same answer!

D. The Factor Theorem:
A polynomial f(x) has a factor (x - k) if and only if f(k) = 0.

In Summary:

The remainder "r", obtained in the synthetic division of f (x) by (x - k) provides the following information:
1. the remainder "r" gives the value of "f" at x = k. That is r = f (k)

2. If r = 0, then (x - k) is a factor of f (x)

3. If r = 0, then (k, 0) is an x-intercept of the graph of f (x).

II. The Rational Zero Test:
relates the possible rational zeros of a polynomial

Rational zero = p/q

where "p" and "q" have no common factors other than 1, "p" is a factor of the constant a₀ and q is a factor of the leading coefficient a_n
polynomial:

f(x) = a_nxⁿ + a_n-1x^n-1 + ... + a₂x² + a₁x + a₀

Possible rational zeros = (factors of constant)/(factors of leading coefficient)

Example 1: f (x) = 4x⁵ - 8x⁴ - 5x³ + 10x² + x - 2

So the possible rational zeros = (1, -1, 2, -2) / (1, -1, 2, -2, 4, -4) so

The possible zeros are : {1, -1, 1/2, -1/2, 1/4, -1/4, 2, -2}

Looking at the graph of the function, we see that the real zeros are:
(-1, 0), (-1/2, 0), (1/2, 0), (1, 0), and (2, 0)

You can also use synthetic Division to eliminate possible zeros:
f (x) = 4x⁵ - 8x⁴ - 5x³ + 10x² + x - 2

try x = -1,

so f(x) = (x + 1)(4x⁴ - 12x³ + 7x² + 3x - 2)


so f (x) = (x - 1)(x + 1)(4x³ - 8x² - 1x + 2)


Therefore f(x) = (x - 1)(x + 1)(x -2)(4x² - 1)

f(x) = (x -1)(x + 1)(x -2)(2x + 1)(2x - 1)

so x = -1, x = 1, x = 2, x = -1/2, x = 1/2

so you can see they are the same answers.

III. Third Test for zeros:

A real Number "b" is an upper bound for the real zeros of "f" if no zeros are greater than "b". Similarily, "b" is a lower bound if no real zeros of "f" are less than "b"

Example 1: f(x) = x⁴ - 4x³ + 15

1. degree is even

2. Leading coefficient is positive

so it rises to the left and rises to the right

3. points (-3, 204), (-2, 63), (-1, 20), (0, 15), (1, 12), (2, -1), (3, -12), (4, 15)

So from the points we chose, the lower bound will be 1 and the upper bound is 4

We can use synthetic division to verify these thoughts:

Let x = -1, you can see that the remainder is 20 and the last row is:

1, -5, 5, -5, 20

Let x = 4, you can see that the remaider is 15 and the last row is:

1, 0, 0, 0, 15

Let x = 1, you can see that the remainder is 12 and the last row is:

1, -3, -3, -3, 12

Therefore we can conclude, given x = c:

1. If c is greater than zero and each number in the last row is either positive or zero, "c" is an upper bound for the real zeros of "f".

2. If c is less than zero and each number in the last row are alternately positive and negative, (zero counts as either positive or negative ), "c" is a lower bound for the real zeros of "f".

Therefore -1 is a lower bound and 4 is an upper bound.

Precalculus 1.5 Inverse Functions

Precalculus 1.5 Inverse Functions:

I. Inverse Functions:
A. Let "f " and "g" be two functions such that
f (g(x)) = x for every "x" in the domain of "g"
and
g( f (x)) = x for every "x" in the domain of "f ".

Under these conditions, the function "g" is the INVERSE of the function "f ":

The function "g" is denoted by f ^-1 (read f-inverse), so:

f ( f ^-1(x)) = x

AND

f ^-1( f (x)) = x

The domain of "f " must be equal to the range of f ^-1
AND
the range of "f " must be equal to the domain of f ^-1

Example:
Given f (x) = {(1,2), (4,5), (6,7)}

then f ^-1 (x) = {(2,1), (5,4), (7,6)}

The graphs of "f " and "f ^-1 are related to each other by the fact the graph of "f ^-1" is a reflection of the graph of "f " in the line y = x.

Example:
If the function f has values: {(1,0), (2,3), (4,7)}

then f ^-1 has values of {(0,1), (3,2), (7,4)}

Example:
Find the inverse of f (x) = 4x + 8

1. let f (x) = y, so by substitution we get

y = 4x + 8

2. Switch the x and y

x = 4y + 8

3. Solve for y

x - 8 = 4y

(1/4) x - 2 = y

4. Now y = f ^-1 so

f ^-1 = (1/4) x - 2

Example: Show that f(x) = (x + 8)/3 and g(x) = 3x - 8 are inverse functions of each other. Recall that f(g(x)) = x and g(f(x)) = x therefore:

f (g (x)) = ( (3x-8)+8)/ 3 = (3x)/3 = x so f(g(x)) = x

g ( f (x)) = 3((x + 8)/3) - 8 = x + 8 - 8 = x so g (f (x)) = x

so these two functions are inverse functions of each other.

Example: Find the inverse function of f (x) = x³+5

1. y = x³ + 5
2. x = y³ + 5
3. x - 5 = y³

(x - 5)^1/3 = y

so

f ^-1(x) = (x - 5)^1/3

Check answer:
f (f ^-1(x)) = x
((x - 5)^1/3)³ + 5 = x - 5 + 5 = x so it checks

II. One - to - one function:
A function is one-to-one if, for "a" and "b" in the domain
f (a) = f (b) implies that a = b.

A function "f " has an inverse function " f " if and only if " f " is one-to-one.

Use the horizontal line test to check if a function is one-to-one. If the equation can have a horizontal line pass through it only once at any value, then the function is one-to-one.

Example: y² = x,
doing the vertical line test (to see if it is a function) you see that when x = 1, y = -1 or y = 1 so
this is not a function
but by using the horizontal line test, the line never hits twice so therefore this equation has a one-to-one relationship.

Example: y = x³
this is a function and it has a one-to-one relationship so therefore
this is a one-to-one function.

Testing for One-to-one Functions:

If the function f (x) = x³ + 7
Show that f (a) = f (b)

a³ + 7 = b³ + 7
a³ = b³
a = b so yes it is.

Example:
g (x) = 5x² + 8

5 (a)² + 8 = 5 (b)² +8

5a² = 5b²

a² = b²

so you could get:
a = b or
-a = b or
a = -b or
-a = -b

so therefore this function is not one-to-one.

Precalculus 1.2b Graphs of Functions

Precalculus 1.2 b Graphs of functions:

I. to graph a piece-wise function:
Given:


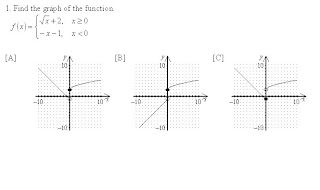
So to find the answer to this, find f(0).
Since 0 is equal to 0, we would use the first function.
so f(0) = √0 + 2 = 2, this means it is a solid dot at (0, 2) so the answer cannot be C.
and it is an open dot at f(0) = -x - 1 = -1 because it does not equal zero here.

Now we look at the graph of each function.
f(x) = -x - 1 has a negative slope so the answer would be A.

A way to check your answer is by using your graphing utility.

In y₁ = (√ x + 2)(x≥0)

In y₂ = (- x - 1)(x is less than 0)

with this you can see that the graph could be A or C. Now using your table, you can see that
y₁ = 2 where y₂ = 0 (this zero means no - the function doesn't exist here) so this means that
y₁ would be a solid dot and y₂ is open.

II. Even Function:

A function f is even if, for each x in the domain of f, f (-x) = f (x)

Example:
f (x) = x² + 4

Test the function:
1st - Is it a function - yes it is
2nd - does f (-x) = f (x)

f (-x) = (-x)² + 4
= x² + 4

Therefore: f (-x) = x² + 4 and
f (x) = x² so
f (-x) = f (x) so this function is even.

III. Odd Function

A function f is odd if, for each x in the domain of f,
f (-x) = - f (x)

Example:
f(x) = x⁵ - x³

f(-x) = (-x)⁵ - (-x)³
= -x⁵ - ^- x³
= - (x⁵ - x³)

-f(x) = - (x⁵ - x³) so...

f (-x) = - f(x) so this function is odd.

IV. Neither an Even Function or Odd Function

A function f is neither if, for each x in the domain of f

a) f (-x) ≠ f (x) AND
b) f (-x) ≠ - f(x)

Example:
f (x) = 5 - 3x

f (-x) = 5 - 3(-x) = 5 + 3x

-f(x) = - (5 - 3x) = -5 + 3x

so since f (x) ≠ f (-x) and
f (-x) ≠ - f(x), this function is neither even or odd

V. Graphically showing even, odd or neither functions:

1. A function whose graph is symmetric with respect to the y-axis is an even function.

2. A function whose graph is symmetric with respect to the origin is an odd function.

3. A function whose graph is not symmetric with respect to the y-axis or the origin is a neither function.

Example:

Can you figure out which of these graphs are even, odd or neither?

1. Graph A is neither because it is not symmetric to the y-axis or the origin.

2. Graph B is an even function because it is symmetric to the y-axis.

3. Graph C is an odd function because it is symmetric to the origin.

VI. The Greatest Interger Function:

is denoted by [[x]] and is defined by

f (x) = [[x]] = the greatest integer less than or equal to x.

The graph of the greatest integer function jumps vertically one unit at each integer and is contant (a horizontal line segment) between each pair of consecutive integers.

Example: f (x) = [[x]]

[[-2]] = -2

[[-1.5]] = -2

[[-0.5]] = -1

[[1.5]] = 1

From this you can see that the value of the x rounded down to the nearest integer.

To put this on the graphing utility:

y₁ = math → num↓#5 int( x)

Example 2:

f (-4) = [[-1]] - 2 = -1 - 2 = -3

f (-3.5) = [[-.875]] - 2 = -1 - 2 = -3

So we can definitely throw C away. So looking at A or B,

and since f (-4) = -3, this means the dot is closed so the answer would be A.

Precalculus 2.2 Polynomial Functions of Higher Degree

Precalculus 2.2 Polynomial Functions of Higher Degree

You can find extra notes at the following website:

http://scidiv.bcc.ctc.edu/FL/MATH105/sso0202.pdf

I. Graphs of:

Polynomial functions are continuous if -
they have no breaks, holes, or gaps.

Example 1: This graph is continuous

Example 2: Piece-wise functions are not continuous

II. Simple Graphs:
f (x) = xⁿ, where n is greater than zero

1. If n is even, the graph of y = xⁿ touches the axis at the x-intercept.
2. If n is odd, the graph of y = xⁿ crosses the axis at the x-intercept.

III. The Leading Coefficient Test
Given f(x) = a_nxⁿ + ... + a₁x + a₀

1. When "n" is odd:
a. If the Leading Coefficient is positive (a_n is greater than 0), the graph falls to the left and rises to the right.
b. If the Leading Coefficient is negative (a_n is less than 0), the graph rises to the left and falls to the right.

2. When "n" is even:
a. If the Leading Coefficient is positive (a_n is greater than 0), the graph rises to the left and right.
b. If the Leading Coefficient is negative (a_n is less than 0), the graph falls to the left and right.

*This determines ONLY the right and left behavior of the graph!

IV. Zeros of Polynomial Functions:
1. The graph of "f" has at most "n" real zeros.
2. The function has at most n-1 relative extrema (relative minimum or maximums).

Example: Given y = x²
n = 2
so
2 real zeros and (n-1) or 1 minimum

V. Real Zeros:
1. x = a is a zero of the function "f"
2. x = a is a solution of the polynomial equation f (x) = 0
3. (x - a) is a factor of the polynomial f (x)
4. (a, 0) is an x-intercept of the graph of "f".

Example: f (x) = x² - 8x + 15
0 = x² - 8x + 15
0 = (x - 5)(x - 3)
x = 5 and x = 3

therefore the zeros are (5, 0) and (3, 0)

x² so n = 2 so ... there are at least 2 real zeros
graph: a is greater than 0 so this function rises to the left and right.

Example: f (x) = (-3/8) x⁴ - x³ + 2x² + 5
Given the zeros:

1. Leading Coefficient = -3/8
2. Leading Degree is 4 so it is even
Therefore the graph falls to the left and the right.

Note: use the calculator to find the intercepts if algebraically not able to at this stage

When you graph the above function:
Maximum: (-2.914851686, 19.68779879)
Zeros: (-4.141946129, 0) and (1.934035914, 0)

Example: f (x) = x³ - 4x

Leading Coefficient = 1
Leading degree is 3 so it is odd

The graph falls to left and rises to the right

0 = x³ - 4x
0 = x (x² - 4)
0 = x (x + 2)(x - 2)
0 = x, x = -2, and x = 2

Maximum value (-1.15, 3.08)
Minimum value (1.15, -3.08)

VI. Multiplicity for the Factor (x - r)^k

In general, a factor of (x - r)^k yields a repeated zero x = r of multiplicity k.
1. If k is odd, the graph crosses the x-axis at x = r
2. If k is even, the graph touches (but does not cross) the x-axis at x = r.

Example: f (x) = 4x² -6x + 9

Find all zeros:

0 = 4x² - 6x + 9
0 = (2x - 3)(2x - 3)
0 = (2x - 3)²

so we know a zero is (3, 0)

Using #2 above, k = 2 so it is even, therefore the graph touches the x-axis at x = 3

Example 2: Find the x-intercepts and multiplicity of f (x) = 2(x + 2)²(x - 3)
x-intercepts are (-2, 0) and (3, 0) and the mutiplicity of 2.

2.2b Finding a Polynomial with Given Zeros:

I. Finding a Polynomial with given zeros

Example 1: given zeros: -4 and 5
1. For each of the given zeros, form a corresponding factor.
We have: x = -4 and x = 5

f (x) = (x + 4)(x - 5)
= x² - 5x + 4x - 20
= x² - x - 20

Now sketch the graph:
1. Apply the Leading Coefficient test
Leading Coefficient is 1 and 1 is positive and the degree is 2 so it is even
Therefore the graph rises to the left and right.
2. Use zeros given: (-4, 0) and (5, 0)
3. Find the y-intercept by letting x = 0
f (0) = -20
4. Find the vertex
x = -b/(2a) = 1/2
f (1/2) = -20.25

So now you have 4 points to plot so you can sketch the curve.

Example 2: Zeros: 0, 2, and -1/3 so
x = 0, x = 2, and x = -1/3
f (x) = (x)(x - 2)(3x + 1)
= (x²)(3x + 1)
= 3x³ + x² - 6x² - 2x
= 3x³ - 5x² - 2x

Sketch the graph:
1. Leading Coefficient test - odd and positive so falls to the left and rises to the right
2. Use given zeros which is also the y-intercept

Example 3: zeros: 6 + √ 3 and 6 - √3
Therefore:
x = 6 + √3 and x = 6 - √3

f (x) = (x - 6 - √3)(6 - 6 + √3)
= x² - 6x + x√3 - 6x + 36 - 6√3 - x√3 + 6√3 - (√3)²
= x² - 12x + 36 - 3
= x² - 12x + 33

Example 4: (use grouping)
Zeros: 4, 2 + √7, 2 - √7

f (x) = (x - 4)(x - 2 - √7)(x - 2 + √7)
= (x - 4)(x² - 2x + x√7 - 2x + 4 - 2√7 - x√7 + 2√7 - ( √7)²
= (x - 4)(x² - 4x + 4 - 7 )
= (x - 4)(x² - 4x - 3)
= x³ - 4x² - 3x - 4x² + 16x + 12
= x³ - 8x² + 13x + 12

II. The Intermediate Value Theorem:
- This theorem helps locate the real zeros of a polynomial function.

Example 1: Find a value x = a where a polynomial function is positive and another x = b where it is negative, you can conclude that the function has at least one real zero between the two values.

f (x) = x³ - 3x² + 3

1. Using the leading coefficient test, the graph falls left and rises right
2. Finding a couple of points, (0, 3) is one point and (-3, -5) is another point

(0, 3) is above x-axis while (-3, -51) is below the x-axis so there is a real zero between them (the graph crosses the x-axis)

(2, -1) is also below the x-axis so there is a real zero between (0, 3) and (2, -1)

(5, 53) is another point and that is above the x-axis so there is another real zero between (2, -1) and (5, 53).

This tells us that the graph crosses the x-axis in three different places.

Next using the calculator to find the "exact" zeros:

1. In your calculator: y₁ = x³ - 3x² + 3

2. Press 2nd trace, #2 zero
going from the left, go below the x-axis and this will be your lower bound and then above the x-axis, this will be your upper bound, and then guess around the x-axis.

(-.8793852, 0) which was between (-3, 51) and (0, 3)
(1.3472964, 0) which was between (0, 3) and (2, -1)
(2.5320889, 0) which was between (2, -1) and (5, 53)

Example 2: g(x) = (1/8)(x + 1)² (x - 3)³

1. the highest degree is 5 which is odd and the leading coefficient is positive so the graph falls to the left and rises to the right.

2. There could be 5 different x-intercepts so trying some different points we have
(-2, -15.625), (-1, 0), (0, -3.375), (1, -4), (2, -1.125), (3, 0), (4, 3.125)

From our table, we see that there are only 2 x-intercepts.

Check this algebraically:

0 = (1/8)(x + 1)²(x - 3)³
0 = (1/8)(x + 1)²
0 = x + 1
-1 = x

0 = (x - 3)³
0 = x - 3
3 = x

These are the two points that we found graphically.

Precalculus 2.1 Quadratic Functions

Precalculus 2.1 Quadratic Functions

2.1 Quadratic Functions

You can find extra notes on this website:

http://scidiv.bcc.ctc.edu/FL/MATH105/sso0201.pdf

I. Quadratic Functions
Definition: Let “n” be a non-negative integer and let a_n, a_n-1, …, a₂, a₁, a₀
be real numbers with a_n ≠ 0.

The Function

f (x) = a_nxⁿ + a_n-1x^n-1 + … + a₂x² + a₁x + a₀

is called a POLYNOMIAL FUNCTION of x with degree “n”

Polynomial functions are classified by degree:

f (x) = a is a constant function
f (x) = mx + b is a linear function
f (x) = ax² + bx + c is a quadratic function where a ≠ 0 and {a, b, c } is contained in the set of Real Numbers

Parabola – a graph of a quadratic function is a special type of u-shaped curve.

Since this graph opens upward, given f (x) = ax² + bx + c, "a" is greater than 0.
if "a" is less than 0, then the parabola is opened downward.

Vertex is the turning point and the axis of symmetry is perpendicular through the x-value of the vertex. Can be found by x = -b/(2a)

Key: using f(x) = ax²
if "a" is greater than 1, the graph is a vertical stretch of the graph y= f(x)
if 0 is less than "a" which is less than 1, the graph is a vertical shrink of the graph y = f(x)

Standard Form of a Quadratic Function:

f(x) = a (x - h)² + k, where a ≠ 0,
the axis of symmetry is the vertical line x = h, and the vertex of the function is (h, k)

Example 1: Given the vertex (4, -1) and the point (2, 3), what is the equation of the quadratic function.

y = a (x - h)² + k
3 = a (2 - 4)² + (-1)
4 = a (-2)²
4 = a (4)
1 = a

y = 1 (x - 4)² - 1

Example 2: Given the vertex is (5/2, -3/4) and a point (-2, 4), what is the equation of the quadratic function.

y = a(x - h)² + k
4 = a(-2 - 5/2)² + (-3/4)
4.75 = a(-4.5)²
4.75 = 20.25 a
19/81 = a

y = (19/81)(x - 5/2)² - 3/4

II. Maximum or Minimum values:
To find the maximum or minimum, find the vertex by using
x = -b/(2a)

Example: Given C = 800 - 10x + 0.25 x²
find the minimum cost and the number of fixtures:

x = -b/(2a) = 10/((2)(.25) = 10/.5 = 20

therefore there will be 20 fixtures

C = .25 (20)² - 10(20) + 800
C = 700

So the minimum cost is $700

You can check by graphing, and the minimum point is (20, 700)


III. Identify the vertex and the intercepts Algebraically.

f(x) = x² + 9x + 8

Vertex is x=-b/2a = -9/2 = -4.5

f (-9) = (-4.5)² + 9(-4.5) + 8
= 20.25 - 40.5 + 8
= -12.75

vertex is (-4.5, -12.25)

Intercepts:
Let x = 0
f (0) = 0² + 9(0) + 8 = 8
(0, 8)

Let y = 0
0 = x² + 9x + 8
0 = (x + 8)(x + 1)
x = -8 and x = -1
so
(-8, 0) and (-1, 0)

IV. Find the equation of a quadratic

Given the two x-intercepts (-2, 0) and (10, 0), can you find the equation?

A. if the parabola opens upward, "a" is greater than 0,

y = a (x - p)(x - q)

because there can be lots of answers depending upon what the value of "a", so we will
Let a = 1

f (x) = (x - (-2))(x - 10)
= (x + 2)(x - 10)
= x² - 10x + 2x - 20
= x² - 8x - 20

B. if the parabola opens downward, "a" is less than 0,
y - a (x - p)(x - q)
because again, there can be lots of answers depending upon what the value of "a", so we will
Let a = -1

f(x) = -(x + 2)(x - 10)
= -(x²- 8x - 20)
= -x² + 8x + 20

Precalculus Chapter 1.1b + 1.2a

Chapter 1.1b Functions + 1.2a Graphs of Functions

I. Application: A right triangle is formed in the first quadrant by the x-axis and y-axis and a line through the point (2, 1). Write the area of the triangle as a function of “x”, and determine the domain of the function.

1. Graph the triangle:
- one side of the triangle is the x-axis and another side is the y-axis. Since we do not know exactly where the points of the third line cross the 2 axes, we use the points (0,y) and (x,0).

A = ½ (base)(height) = ½ xy

Since (0, y) , (2, 1) and (x, 0) all lie on the same line, the slopes between any pairs of points are equal.

M = (1 – y)/(2 – 0) = (1 – 0)/(2 – x)

1 – y = 2/(2 – x)

y = -2/ ( 2 – x) + 1

y = (-2 + (2 – x))/ (2 – x)

y = -x / (2 – x)

y = x / (x – 2)

Now using substitution:

A = ½ x (x / (x – 2)) = x²/(2x – 4)

The domain is (2, ∞ ) since the area has to be greater than zero.

II. Evaluating a Difference Quotient:

If f (x) = 2x, then using the difference quotient below

Using f(x) = 2x, plug into the equation above:

2h/h = 2

Example 2: Given 5x – x²,

-5 – h , where h cannot equal zero

Example 3:


Increasing, Decreasing, and Constant Functions

A function “f” is increasing on an interval if, for any x₁ and x₂ in the interval, when x₁ is less than x₂ implies that f (x₁ ) is less than f(x₂ )
A function “f” is decreasing on an interval if, for any x₁ and x₂ in the interval, when x₁ is less than x₂ implies that f (x₁ ) is greater than f(x₂ )
A function “f” is constant on an interval if, for any x₁ and x₂ in the interval, f (x₁ ) = f(x₂ )

Check out this website:
http://www.mathematicshelpcentral.com/lecture_notes/precalculus_algebra_folder/increasing_and_decreasing_functions.htm

Relative Minimum and Relative Maximum:
1. A function value f (a) is called a relative minimum of “f” if there exists an interval that contains a such that:

So you can see that the function is increasing from (- ∞ , -3) and then again from (2, ∞). The function is decreasing from (-3, 2)

Now lets see an example of relative minimum and relative maximum points:


So you can see that the function's relative minimum point from ( - ∞, 0 ) is
"a" while its relative minimum point from ( 0, ∞) is "c" . The relative maximum point is "b".

Precalculus Chapter 1.1a Functions

Precalculus Chapter 1.1 Functions and their Graphs

Definition of a Function: A function “f” from a Set A to a Set B is a relation that assigns to each element “x” in the set A exactly one element “y” in the Set B. The Set A is the domain (or set of inputs) of the function “f”, and the Set B contains the range (or set of outputs).

Example:
Set A contains {1, 2, 3, 4, 5} and Set B contains {a, b, c, d, e }

Let's say that Set A maps to Set B in giving the following ordered pairs:
{(1, a), (2, c), (3, c), (4, d), (5, b)}
The mapping would look like:


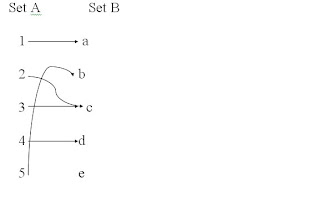
Since every input there is only one output, therefore this is a function.

Remember, for every input there is only one output.





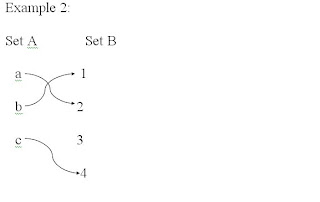
{(a, 2), (b, 1), (c, 4)}

Where a, b, and c are the input and 1, 2, and 4 are the output

CHARACTERISTICS of a FUNCTION:

1). Each element in Set A must be matched with an element of Set B.
2). Some elements in Set B may no be matched with any elements of Set A.
3). Two or more elements of Set A may be matched with the same element of Set B.

FUNCTIONS are commonly represented in four ways:
1). Verbally – by a sentence that describes how the input variable is related to the output variable.
2). Numerically – by a table or list of ordered pairs
3). Graphically
4). Algebraically by an equation in two variables.
example: y = x² where “y” is a function of “x”

“x” is the independent variable (input or domain)
“y” is the dependent variable (output or range)

TESTING FOR FUNCTION REPRESENTED:
1) Algebraically:
a) 2x + y – 6 = 0 Solve for y.
y = -2x + 6
To each value of “x”, there is only one “y” , therefore this example is a function.

b) 3x² + 2y = 1
2y = -3x² + 1
y = (1/2)(-3x² + 1)

Again, this is a function.

c) 4² + 3y² = 6
3y² = -4x² - 6
y² = (1/3)( -4x² - 6)

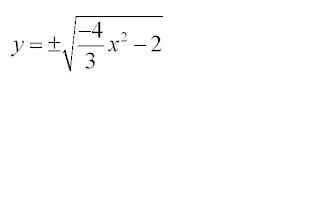
This is not a function!

2). Vertical line test – graph the equation. If you can draw a vertical line and it only touches the graph of the equation once, then the equation is a function. If it touches two or more points, then it is not a function.

3) Function Notation:

Input = x
Output was y, it will now be f(x)

Equation example:
Was: y = 2x + 3
Will now be represented by: f (x) = 2x + 3

EVALUATING A FUNCTION:

Example: f (x) = 2x – 3
f ( 1) = 2 (1) – 3 = 2 – 3 = -1
f (-3) = 2 (-3) – 3 = -6 – 3 = - 9
f (x – 1) = 2 (x – 1) – 3 = 2x – 2 – 3 = 2x – 5

Example: g (y) = 7 – 3y
a. g (0) = 7 – 3(0) = 7
b. g (7/3) = 7 – 3 (7/3) = 7 – 7 = 0
c. g ( s + 2) = 7 – 3 (s + 2 ) = 7 – 3s – 6 = 1 – 3s

A PIECEWISE-DEFINED FUNCTION
- this means that the function is defined by two or more equations over a specified domain.

f (x ) =

{ 2x + 1, x ≤ 0

{ 2x + 2, x is greater than 0

Evaluate: f ( -1)

because -1 is less than 0, we use 2x + 1 so

f (-1) = 2 (-1) + 1 = -2 + 1 = -1

Evaluate: f (0 ) because 0 is equal to 0, we use 2x + 2 so

f (0) = 2x + 2 = 2 (0) + 2 = 2 f (2) = 2x + 2 = 2 (2) + 2 = 4 + 2 = 6

Precalculus Prerequisite 5 - Inequalities

Precalculus Prerequisite Section 5 - Inequalities:


II. Solving Inequalities Algebraically and Graphically:
A. Solve the Inequality:

Precalculus P4.b Completing the square and

I. Completing the Square:

if x² + bx = c, then

x² + bx + (b/2)² = c + (b/2)²

so:

(x + b/2)² = c + (b²)/4

Example:

x² + 4x - 32 = 0

x² + 4x = 32

therefore b = 4 so:

x² + 4x + (4/2)² = 32 + (4/2)²

(x + 2)² = 32 + 4

(x + 2)² = 36

take the square root of both sides:

x + 2 = ± √36

x + 2 = ±6

x = -2 ±6

so x = 4 and x = -8

Now check your answers:

(-8)² + 4(-8) - 32 = 0

64 - 32 - 32 = 0

0 = 0

(4)² + 4(4) - 32 = 0

16 + 16 - 32 = 0

0 = 0

They both work so our answers are x = 4 and x = -8

Example 2:

x² - 2x - 3 = 0

x² - 2x = 3

x² - 2x + (-2/2)² = 3 + (-2/2)²

x² - 2x + (-1)² = 3 + 1

(x - 1)² = 4

x - 1 = ±√4

x = 1 ±2

x = 1 + 2 = 3

x = 1 - 2 = -1

check answers:

(3)² - 2(3) - 3 = 0

9 - 6 - 3 = 0

0 = 0

(-1)² - 2(-1) - 3 = 0

1 + 2 - 3 = 0

0 = 0

II. Quadratic Formula:

if ax² + bx + c = 0 then

Example:

9x² - 12x - 14 = 0

x = (12 ± √(144 - 4(9)(-18)))/(2(9))

x = (12 ± √648)/18

x = (12 ± √324 √2)/18

x = (12 ± 18 √2)/18

x = (2 ± 3 √2)/3

x = 2/3 ± √2

III. Solving an Equation of Quadratic Type:

x⁴ + 2x³ - 8x - 16 = 0 by grouping

x³ (x + 2) - 8(x + 2) = 0

(x³ - 8 ) ( x + 2) = 0

x³ = 8

x = 2

x + 2 = 0

x = -2

now check the answers:

they both check!

IV. By factoring more difficult equations:

4x²(x - 1)^1/3 + 6x (x - 1)^4/3 = 0

2x [2x (x - 1)^1/3 + 3 (x - 1)^4/3] = 0

remember that (x - 1)^4/3 = (x - 1) ^3/3 (x - 1) ^1/3

and 3/3 = 1 so:

2x(x - 1)^1/3 [2x + 3 (x - 1)^3/3 ] = 0

2x(x - 1)^1/3 [ 2x + 3x - 3] = 0

2x(x - 1)^1/3 [5x - 3] = 0

so:

2x = 0

x = 0

(x - 1)^1/3 = 0

x - 1 = 0

x = 1

5x - 3 = 0

5x = 3

x = 3/5

check your answers!

V. Solving an equation involving a radical:

√(-5x + 4) - x = 6

√(-6x + 4) = x + 6

now square both sides:

(√(-6x + 4))² = (x + 6)²

-6x + 4 = x² + 12x + 36

0 = x² + 18 x + 32

0 = (x + 16)(x + 2)

x = -16 and x = -2

check both answers:

√(-6 (-16) + 4) - (-16) = 6

√(96 + 4 ) + 16 = 6

10 + 16 = 6

not true

√(-6(-2) + 4) - (-2) = 6

√(12 + 4) + 2 = 6

√16 + 2 = 6

4 + 2 = 6

this one works! so

x = -2 is the only answer!

VI. Solving an Equation Involving Two radicals:

√x + √(x - 20) = 10

√ x = 10 - √(x - 20)

(√x)² = (10 - √(x - 20))²

x = 100 - 20√(x - 20) + (x - 20)

0 = 80 - 20√(x - 20)

-80 = -20√(x - 20)

4 = √(x - 20)

4² = x - 20

16 = x - 20

36 = x

Now check your answer.

√36 + √(36 - 20) = 10

6 + 4 = 10 so it checks

VII. Solving an Equation with Rational Exponents

Example:

(x - 5)^2/3 = 16

to undo exponents, multiply by the reciprocal

((x - 5)^2/3)^3/2 = 16^3/2

x - 5 = 64

x = 69

Check your answer.

(69 - 5)^2/3 = 16

64^2/3 = 16

16 = 16 so it checks

VIII. Solving an Equation Involving Absolute Value:

recall: |x| = 9

x = 9 and x = -9 so

|3x + 2 | = 7

3x + 2 = 7 and 3x + 2 = -7

3x = 5 and 3x = -9

x = 5/3 and x = -3

Precalculus Prerequisite 4a - Solving Equations Algebraically and Graphically

Prerequisite 4a - Solving Equations Algebraically and Graphically

I. Vocabulary:

A. An Equation - is a statement that two algebraic expressions are equal.
Example: 2x + 4 = 10

B. To Solve an equation in "x" means to find all values of "x" for which the statement is true. These values are solutions.
Example: 2x + 4 = 10
2x = 6
x = 3

C. An identity equation- an equation that is true for every real number in the domain of the variable.
Example: x² - 6x + 9 = (x - 3)²

D. Conditional Equation - an equation that is true for just some (or even none of the real numbers in the domain of the variable.
Example 1: x² - 6x + 9 = 0
(x - 3)² = 0
x = 3 only

Example 2: 3x + 2 = 4x - 5
x = 7 only

Example 3: x² + 3x + 4 = 4x - 5
no solutions

E. Notations:
1. all real numbers - R
2. no solutions - { } empty set or Æ is the null set

F. Solving an Equation Involving Fractions:
Example 1: x/4 + (2x)/3 = 6

multiply by the LCD
4, 3 are the denominators so the LCD = 12, so multiply each term by 12:

12(x/4) + (12)(2x)/3 = (12)(6)
3x + 8x = 72
11x = 72
x = 72/11

Example 2: 5/x + (3x)/2 = 7

LCD = 2x

(2x)(5/x) + (2x)(3x)/2 = (2x)(7)
10 + 3x² = 14x
3x² - 14 x + 10 = 0

use the quadratic equation

x = (-b ± Ö(b² - 4ac))/(2a)

x = (14 ± Ö(14² - (4)(3)(10)))/((2)(3))

x = (14 ± Ö76)/3

x = (7 + Ö 19)/3 and x = (7 - Ö19)/3

or

x = 3.786299648 and x = .8803670188 (check to make sure they both work - they do!)

You can also check by using your graphing calculator -
put in
y₁ = 5/x + (3x)/2 and
y₂ = 7

calc ® intersect, shows the same answers!

G. Extraneous Solutions - an answer or solution that does not satisfy the original equation.
Example:

6/x - 2/(x+3) = (3(x+5))/(x(x + 3))

LCD = x(x+3)

6(x + 3) - 2x = 3(x + 5)
6x + 18 - 2x = 3x + 15
4x + 18 = 3x + 15
x = -3

substituting x=-3 back into the original equation, we have
-2 - 2/0 = 6/0
This is impossible so there is not a solution

H. To find the x-intercepts (a, 0), let y = 0 and solve for x.
To find the y-intercepts (0, b), let x = 0 and solve for y.

Example:
2x² - 5x + 2 = y

x-intercepts, let y = 0
2x² - 5x + 2 = 0
(2x -1)(x - 2) = 0
2x - 1 = 0 and x - 2 = 0
x = 1/2 and x = 2
(.5, 0) and (2, 0)

y-intercepts, let x = 0
2(0)² - 5(0) + 2 = y
2 = y
(0,2)

I. Finding Solutions Graphically:
24x³ - 36x + 17 = 0

graph this on your calculator and you see there is only one solution:
window:
x-values: -10 to 10
y-values: -5 to 40
You can see it is hard to tell how many times the equation crosses the x-axis
so change the y-values to -3 to 3 and you see it crosses y=0 only once

x = -1.414486, y=0

J. Can find answers more than one way, here are a couple using the calculator:
1. y₁ = 24x³ - 36x + 17
2nd trace ® zero
enter left-bound before x=-2 and then right-bound after x=-1, then guess about -1.5, gives the answer.
2. y₁ = 24x³ - 36x + 17
y₂ = 0
2nd trace, #5 intersect, enter 3 times about where the two equations intersect.

K. Remember with points of intersection, always look for all solutions:
Example: 24x³ - 36x + 17 = 2x + 5 has how many solutions?

(-1.393598, 2.212803)
(.3407855, 5.681571)
(1.052813, 7.105626)

remember there can be no points of intersection (therefore no solutions), one solution or many solutions.

L. Solving Polynomial Equations Algebraically:

1. First degree equation - linear equation -
example: 2x + 4 = 7

2. Second degree equation - quadratic equation -
example: 2x² + 4x + 6 = 0

3. Third degree equation - Cubic equation -
example: 2x³ + 2x² + 5x + 2 = 0

4. Fourth degree equation - Quartic equation -
example: 2x⁴ + 3x³ - 2x² + 3x + 2 = 0

5. Fifth degree equation - Quintic equation -
example: 4x⁵ + 2x⁴ - 3x³+ 2x² + x - 4 = 0

Precalculus - Prerequisite 1 - Graphical Representation of Data, P2 - Graphs of Equations, P3 -Lines in a Plane

Prerequisite 1 - Graphical Representation of Data

I) Vocabulary -
A) The Cartesian Plane - the rectangular coordinate plane that represents ordered pairs fo real numbers by points in a plane.
1) x - axis: the horizontal real number line
2) y - axis; the vertical real number line
3) origin - the point of intersection of the x-axis and the y-axis
4)Quadrants - the two axes divide the plane into four parts - the upper right quadrant is quadrant I, the upper left quadrant is quadrant II, the lower left quadrant is quadrant III, and the lower right quadrant is quadrant IV. They go counterclockwise from the positive side of the x-axis.
5) Ordered pair - each point in the plane corresponds to an ordered pair of real numbers (x, y)
called the coordinates of the point.
a) x-coordinate: represents the directed distance from the y-axis to the point
b) y-coordinate: represents the directed distance from the x-axis to the point.
B) Representing Data Graphically:
1) Scatterplot
2) bar graph
3) line graph
4) histogram
5) Interpreting a model based upon the data:
a) look at the data and know how to find the best-fit line
C) Distance Formula: d = √((x₁ - x₂)² + (y₁ - y₂)²)
this is derived from the pythagorean theorem, where the difference of the x's is represented by "a" and the difference of the y's is represented by "b" and so
AB = a² + b² = c²
Example: Given points (-2, -5) and (-3, 4), what is the distance between the two points.
√((-2 - ^-3)² + (-5 - 4)²) =
√((1)² + (-9)²) =
√(1+81)=
√82
E) Midpoint Formula: the mean, or average, of the x-coordinates and the y-coordinates.therefore the midpoint formula is: ((x₁+ x₂) /2, (y₁+ y₂) /2)
example: Given A(-1, 7) and B(3, -3) what is their midpoint?((-1 + 3)/2, (7 + -3)/2) = (2/2, 4/2) = (1, 2)
F) Standard equation of a circle with radius r and center (h, k) is:
r² = (x - h)² + (y - k)²
OR
r = √((x - h)² + (y - k)²)

Prerequisite 2: Graphs of Equations
I) Vocabulary:
A) Solution Point - for an equation in variables x and y, a point (a, b) is a solution point if the substitution of x = a and y = b satisfies the equation.
1) Graph of the equation - the set of all solution points of an equation.
B) How to Sketch the Graph of an Equation by Point Plotting
1) If possible, rewrite the equation so that one of the variables is isolated on one side of the equation.
2) Make a table of several solution points (usually 5 - 7)
3) Plot these points in the coordinate plane.
4) Connect the points with a smooth curve.
C) Using a Graphing Utility to Graph an Equation - to graph an equation involving x and y on a graphing utility, use the following procedure.
1) Rewrite the equation so that "y"is isolated on the left side.
2) Enter the equation into a graphing utility.
3) Determine a viewing window that shows all important features of the graph.
4) Graph the equation.
D) Throughout this course, you will learn that there are many ways to approach a problem.
1) a numerical approach: construct and use a table.
2) a graphical approach: draw and use a graph.
3) an algebraic approach: use the rules of algebra.

Prerequisite 3: Lines in the Plane
I) Vocabulary:
A) the slope of a line - represents the number of units a line rises or falls vertically for each unit of horizontal change from left to right.
Slope: (nonvertical line): Ratio of the vertical change (the rise, Δ y) to the horizontal change (the run, Δ x)
Formula: slope = m = (Δy/Δx) = (y₂ - y₁)÷ (x₂ - x₂)

II) Postulate:
A) In the coordinate plane, 2 nonvertical lines are parallel if and only if they have the same slope. Any two vertical or horizontal lines are parallel.
B) Slope- Intercept Form of the Equation of a Line: y = mx + b where m is the slope of the line and b is the y-intercept (where it crosses the y-axis so point (0,b)
C) Point-slope form of an equation of the line that passes through the point (x₁, y₁) and has a slope of m is
y - y₁ = m(x - x₁)
D) Summary of Equations of Lines
1. General form: Ax + By + C = 0
2. Vertical line: x = a
3. Horizontal line: y = b
4. Slope-intercept form: y = mx + b
5. Point-slope form: y - y₁ = m(x - x₁)
E) Parallel Lines - two distinct non-vertical lines are parallel if and only if their slopes are equal.
F) Perpendicular Lines - two non-vertical lines are perpendicular if and only if their slopes are negative reciprocals of each other. If m₁ = a/b then m₂ = -b/a.
The product of the two slopes are -1. (a/b)(-b/a) = -1
m₁ - -1/(m₂)
Example: if m₁ = 2/3 then
m₂ = -3/2

Geometry Chapter 9.5 Trigonometric Ratios

Chapter 9.5 Trigonometric Ratios:

I) Vocabulary:
A) A Trigonometric Ratio - is a ratio of the lengths of two sides of a right triangle.
1) Sine - abbreviated sin
2) Cosine - abbreviated cos
3) Tangent - abbreviated tan

check out: http://www.tpub.com/math1/20b.htm

Let ΔABC be a right triangle. The sine, the cosine, and the tangent of the acute angle ∠A are defined as follows:

The side opposite angle A = side a
The side opposite angle B = side b
The side opposite angle C = side c = hypotenuse

Sin A = (side opposite angle A)/hypotenuse = a/c

Cos A = (side adjacent angle A)/hypotenuse = b/c

Tan A = (side opposite angle A)/(side adjacent angle A) = a/b

Example: Given ΔABC with AB = 13, AC = 5 and BC = 12 with right ∠C, find the 3 different ratios for angle A and angle B:
Sin A = 12/13
Cos A = 5/13
Tan A = 12/5

Sin B = 5/13
Cos B = 12/13
Tan B = 5/12

Do you notice a pattern?
The Sin A = Cos B and Cos A = Sin B plus the tangents are just reciprocals of each other. This is because the Sine and Cosine are cofunctions of each other.

Example: Find the sine, cosine and tangent of 30°, 45° and 60°.
Sin 30° = 1/2
Cos 30° = √(3)/2
Tan 30° = √(3)/3

Sin 45° = √(2)/2
Cos 45° = √(2)/2
Tan 45° = 1

Sin 60° = √(3)/2
Cos 60° = 1/2
Tan 60° = √3

B)Angle of Elevation - the angle that your line of sight makes with a line drawn horizontally.
Example: The angle of elevation from the base of a water slide to the top of a waterslide is about 13°. The slide extends horizontally about 58.2 meters. Find the vertical height of the slide.

1) Draw a diagram. Place the appropriate values where they belong.
2) Using the trigonometric functions, find the one you need.
a) We need to know the side opposite the angle and we know the side adjacent to the angle so this would be tangent.
b) Tan 13° = h/58.2
h = 58.2 (tan 13°)
h = 13.43652872 meters

Chapter 9.6 Solving Right Triangles:

I) Vocabulary:
A) Solve a right triangle - to determine the measures of all six parts, 3 sides and 3 angles.
Example: Given ΔABC, angle C is the right angle, angle A = 26°, AB = 4.5 inches, find AC, BC and angle B.
measure of angle A = 26°
measure of angle B = ?
measure of angle C = 90°
AB = c = 4.5 inches
BC = a = ?
AC = b = ?

A triangle has 180° so 26°+ 90° = 116°, 180° - 116° = 64° = measure of angle B

From the angle A, we know the hypotenuse or side "c" = 4.5, to find side "a", this is opposite to angle A so we would use sine function.
sin 26° = a/4.5
a = 1.972670161 inches

From the angle A, we know the hypotenuse and need to find side "b", this is adjacent to angle A so we would use the cosine function.
cos 26° = b/4.5
b = 4.044573208 inches

Therefore we have now solved the triangle:
measure of angle A = 26°
measure of angle B = 64°
measure of angle C =90°
a = 1.972670161 inches
b = 4.044573208 inches
c = 4.5 inches